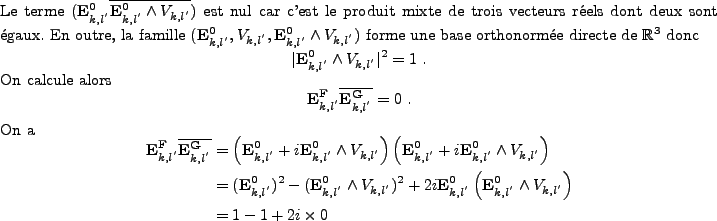Définissons d'abord les fonctions
<I>E'kl et
<I>H'kl (II.8.4) qui d'après la définition 14 donnent les
fonctions de base
![]() par (II.8.5). Pour cela introduisons les ondes planes de polarisations complexes que nous appellerons ``fonctions de
type <I>F ou de type <I>G''.
par (II.8.5). Pour cela introduisons les ondes planes de polarisations complexes que nous appellerons ``fonctions de
type <I>F ou de type <I>G''.
| (II.8.24) |  |
Un calcul élémentaire montre que l'on a la proposition 12 suivante.
| (II.8.27) |  |